Hillbert paradox
Can we travel in past and what is grand father paradox?
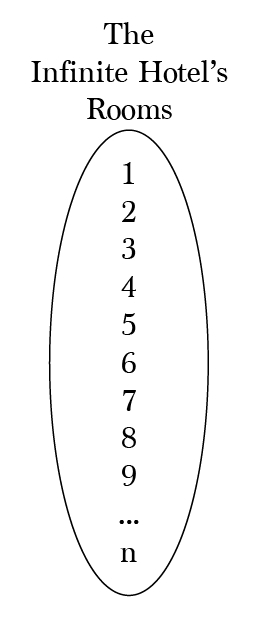
Let n=room no. and if he transferred all infinite people to n+1 room no. of hotel then one room of hotel became left. After some time a bus came in which infinite people are there then tomorrow became worried but he got a idea. He thought if n=room no. of current people if he multiply it by 2 so n×2=even no.
So if he transferred infinite people in even room number.so odd number became left and it will occupied by bus passenger then tom goes to sleep after half and hour infinite bus come in which infinite people are there and they want room in hotel . Tom get worried because he will lose infinite money if he do not arranged rooms for them then he got an idea. He thought that in ancient time greek Mathematician Euclid proved
that prime number are also Infinite. So if n=room no then if he shift all his existing customers to the power of their room no for eg-if n=3 (room no.) So he will be shifted to 2^3 =8(room number) then if he take prime no 3 and from this number he give room number to 1st bus passenger according to their seat number for eg let n=5(seat number)
So if the passenger will go to room no 3^5 =243 room number and this process will gi till infinite passenger don't get their room.
Here we only take example of infinite natural number but what happen if we take example of rational number. At that time it confused all the people. But in 19 th century German mathematician georg
Cantor give that if we write rational number in one and one correspondence then we can alot room in hotel.
All the people believe that in hilbert grand Hotel is really infinite but Georg cantor say that how we alot real number room no for this. He gave birth to his cantor's diagonal proof to proof.
First of all we have to know that what is time?
Time is the indefinite continued progress of existence and events that occur in an apparently irreversible succession from the past, through the present, into the future.
Some of the scientists believe that it is our 4th dimension and time travel is possible but there are numerous of paradox one such paradox is grandfather paradox- In this paradox let carry is a scientist and with his hard work. He made a time machine . He had a very bad relationship with his grandfather so he wanted to kill his grandfather. So he take his time machine and went to that time when his
Grandfather was not married. He then killed his grandfather. So how he exist because if his grandfather and grandmother don't marry each other then how his father born and if his father don't born then how carry born.
What is Hilbert grand Hotel paradox?
Hilbert's paradox of the Grand Hotel (colloquial: Infinite Hotel Paradox or Hilbert's Hotel) is a thought experiment which illustrates a counterintuitive property of infinite sets. Let's understand this suppose that there is hotel in which infinte rooms are there in which infinite people live. The name of hotel manager is tom. If one new guest come how can tom manage here in his mind one idea and the idea is
Let n=room no. and if he transferred all infinite people to n+1 room no. of hotel then one room of hotel became left. After some time a bus came in which infinite people are there then tomorrow became worried but he got a idea. He thought if n=room no. of current people if he multiply it by 2 so n×2=even no.
So if he transferred infinite people in even room number.so odd number became left and it will occupied by bus passenger then tom goes to sleep after half and hour infinite bus come in which infinite people are there and they want room in hotel . Tom get worried because he will lose infinite money if he do not arranged rooms for them then he got an idea. He thought that in ancient time greek Mathematician Euclid proved
that prime number are also Infinite. So if n=room no then if he shift all his existing customers to the power of their room no for eg-if n=3 (room no.) So he will be shifted to 2^3 =8(room number) then if he take prime no 3 and from this number he give room number to 1st bus passenger according to their seat number for eg let n=5(seat number)
So if the passenger will go to room no 3^5 =243 room number and this process will gi till infinite passenger don't get their room.
Here we only take example of infinite natural number but what happen if we take example of rational number. At that time it confused all the people. But in 19 th century German mathematician georg
Cantor give that if we write rational number in one and one correspondence then we can alot room in hotel.
